Quantum basics explained with a billiards example

Quantum basics explained with a billiards example. How quantum state, measurement and entanglement would look like in physical world?

The blog article tries to explain quantum fundamentals in an understandable way. Apologies to real quantum experts about cutting some corners!

What qubits are?

To simplify it a lot, entities that have quantum mechanical characteristics.

Some examples about qubits in quantum computers are atoms, ions, electrons and photons.

In the following examples, you can think a qubit as a billiard ball.

Quantum state

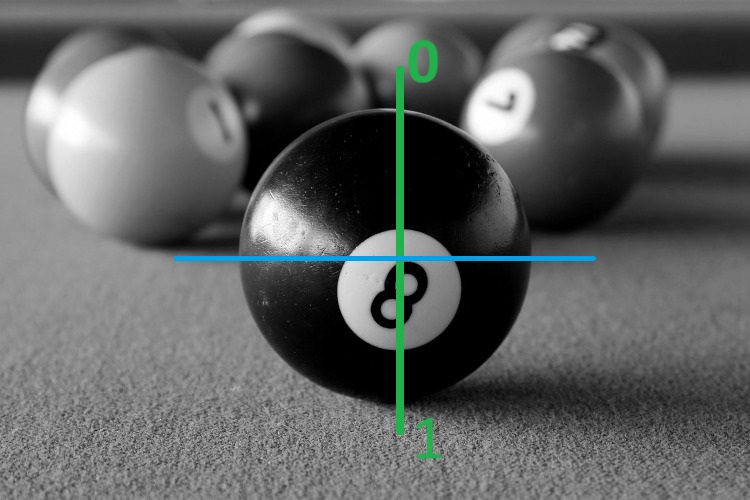
Assume you roll the eight-ball on the billiards table in a dark room. At any given moment the number eight is pointing to some direction. Maybe exactly up, exactly down or something in between.

In quantum billiards the direction is known as state.

State is said to be 0, if the number points to the roof. And 1, if the number is facing down. These basis states can be achieved only by manipulating the ball or the environment.

In practice the ball in a completely dark room is in superposition if there are no external interference. Unlike some learning sources claim, superposition does not mean that the number would face both up and down simultaneously.

Rather, superposition can expressed as a combination of probabilities of the number pointing up or down. They always add up to 100 %.

Soo… What are those probabilities?

A ball rolling randomly in perfectly clean room both up and down direction has equal probability. 50 % to point up, 50 % to point down.

It would be possible to manipulate those probabilities. For example by adjusting the mass center of the billiard ball could make the chances 30 % up, 70 % down.

Measuring the state

State without measurement is useless. Who cares where the ball is pointing in the pitch-black room?

It’s like Schrödinger’s cat being in the box. Does not make a difference to anybody until somebody checks whether it’s alive.

Even though the state is a fuzzy concept, the state is never random! We just don’t know the result before measuring it.

Should be easy to do check! Just turn on the lights in the game room. In regular billiards it would be easy to express the precise direction of the ball by rotation angles.

Quantum billiards works a bit differently. When the lights are turned on, you can only see if the label is pointing up or down.

Let’s say it’s pointing up when the lights are turned on after the first roll.

You reset the state by switching off the lights and rolling the ball again around the table.

Lights are turned on for the second measurement. Now the number is pointing down. In the next experiment also down. Then up again.

After 1000 experiments, the results would be around 50 % up and 50 % down for a flawless ball.

Quantum computers are probabilistic. They are able to manipulate the qubits to known probabilities to perform calculations.

Entanglement

Qubit’s state is not meaningful without measurement. Neither does measuring a single qubit make any useful computation.

Unless you do something as silly as Google and generate a bunch of useless random numbers.

Typically multiple related qubits are required to calculate something. That’s where entanglement comes in.

Entanglement means that two or more qubits behave as a single system. The phenomen is part of the qubit’s state.

There are many ways in which the qubits can be entangled. For example:

• Two qubits always produce the same result
• Two qubits always produce different results
• Exactly one of three qubits is measured as 1
• Previous examples behaving probabilistically
• And much more…

Some learning resources imply that qubits in the entanglement are exact clones of each other. It would be same as having a set of dice that each give the same result on single roll. How could you do anything meaningful with that?

Better expression is that the entangled qubits behave like a single system. One qubit in such setup cannot be described without the others.

It would like installing small magnets inside two billiard balls to make their state “entangled”. Every time they are rolled, they attach to each other so that they always point to opposite directions. Their states and measurements are not either independent or full clones. It is better to say they are dependent on each other.

Spookiness of entanglement

The spooky part is that the physical distance of the qubits is insignificant in entanglement. The particles could be entangled even in opposite corners of the universe.

According to my understanding entanglement conflicts with general theory of relativity which assumes that nothing can travel faster than light. Because in entanglement the communication is instant.

Even Einstein could not explain how this is possible and he famously quoted entanglement being “spooky action at a distance”. Regardless of physics theories falling short with entanglement, there exists re-producible experiments proving that communication really happens.